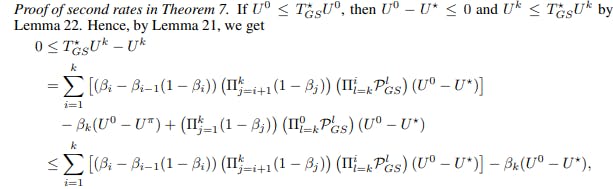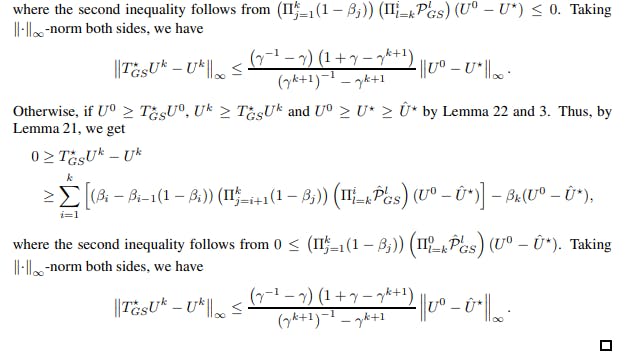Making Sense of AI Learning Proofs | HackerNoon

Abstract and 1 Introduction

1.1 Notations and preliminaries

1.2 Prior works

2 Anchored Value Iteration

2.1 Accelerated rate for Bellman consistency operator

2.2 Accelerated rate for Bellman optimality opera

3 Convergence when y=1

4 Complexity lower bound

5 Approximate Anchored Value Iteration

6 Gauss–Seidel Anchored Value Iteration

7 Conclusion, Acknowledgments and Disclosure of Funding and References

A Preliminaries

B Omitted proofs in Section 2

C Omitted proofs in Section 3

D Omitted proofs in Section 4

E Omitted proofs in Section 5

F Omitted proofs in Section 6

G Broader Impacts

H Limitations

F Omitted proofs in Section 6

Next, we prove following key lemma

Proof of Lemma 21. First, we prove first inequality in Lemma 21 by induction.

If k= 0,

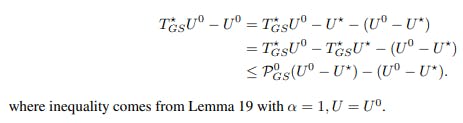
By induction,

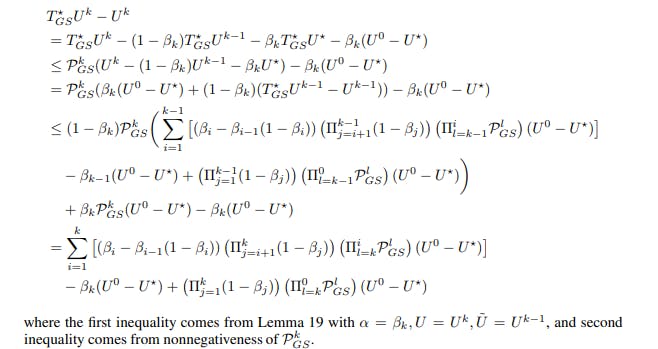
First, we prove second inequality in Lemma 21 by induction.

If k= 0,

By induction.

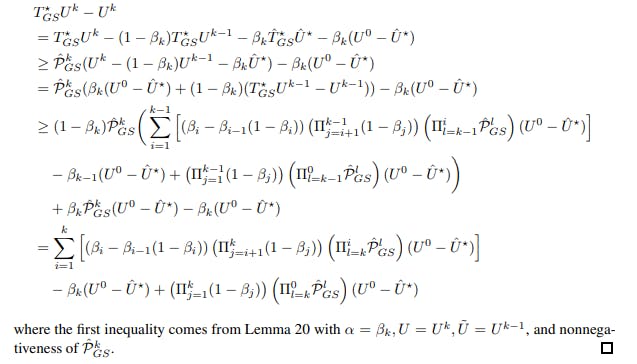
Now, we prove the first rate in Theorem 7.

For the second rates of Theorem 7, we introduce following lemma.

Now, we prove the second rates in Theorem 7.